On the dot paper below, draw different squares. Join the midpoints of the sides of each of these squares (in order) to create a new quadrilateral. The first one is shown as an example.
Observe each of the new quadrilaterals formed, and complete the following:
The quadrilateral formed by joining the midpoints of sides of a square is a ________
Task 2
Suppose you were to join the midpoints of sides of a rectangle in a similar fashion. What shape do you think you might get? Think about it, and write your conjecture here:
The quadrilateral formed by joining the midpoints of sides of a
___________________________________ is a _________________________________
Task 3
Now verify your conjecture by drawing different rectangles on the dot paper below and joining the midpoints of the sides.
Think about this!
Explain why you think your conjecture is true (or false)
Task 4
Based on Task 3 does your conjecture hold? If not how would you modify it?
Now make similar conjectures about other special quadrilaterals - rhombus and parallelogram, and verify them. Write your conjectures in the space provided, and use the dot grid for verifying.
GEOMETRIC REASONINGActivity 1: Midpoint Explorations
Task 1
On the dot paper below, draw different squares. Join the midpoints of the sides of each of these squares (in order) to create a new quadrilateral. The first one is shown as an example.
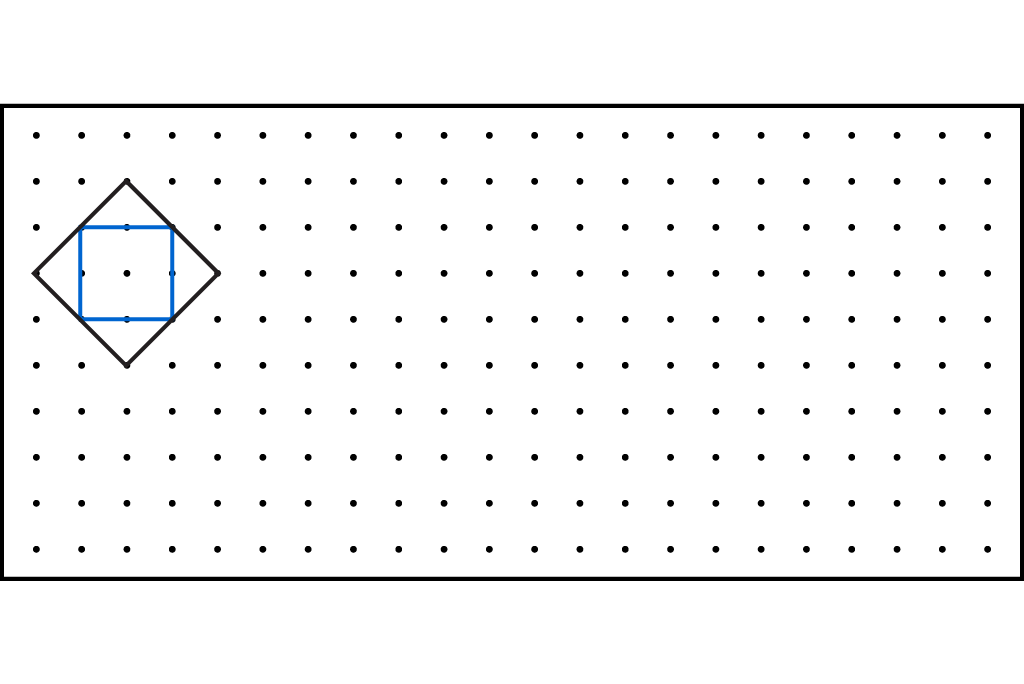
Observe each of the new quadrilaterals formed, and complete the following:
The quadrilateral formed by joining the midpoints of sides of a square is a ________
Task 2
Suppose you were to join the midpoints of sides of a rectangle in a similar fashion. What shape do you think you might get? Think about it, and write your conjecture here:
The quadrilateral formed by joining the midpoints of sides of a
___________________________________ is a _________________________________
Task 3
Now verify your conjecture by drawing different rectangles on the dot paper below and joining the midpoints of the sides.
Task 4
Based on Task 3 does your conjecture hold? If not how would you modify it?
Task 5
Now make similar conjectures about other special quadrilaterals - rhombus and parallelogram, and verify them. Write your conjectures in the space provided, and use the dot grid for verifying.
Conjecture 1:
Conjecture 2:
Task 6
Drawing on your observations in the 5 previous tasks, make a conjecture about the shape formed by joining the midpoints of sides of any quadrilateral.
Extended Task: 1
If possible, draw a quadrilateral, joining whose midpoints of sides in order gives a figure that is NOT a parallelogram. If not possible, explain why.